Jaynes–Cummings–Hubbard model

The Jaynes–Cummings–Hubbard (JCH) model is a many-body quantum system modeling the quantum phase transition of light. As the name suggests, the Jaynes–Cummings–Hubbard model is a variant on the Jaynes–Cummings model; a one-dimensional JCH model consists of a chain of N coupled single-mode cavities, each with a two-level atom. Unlike in the competing Bose–Hubbard model, Jaynes–Cummings–Hubbard dynamics depend on photonic and atomic degrees of freedom and hence require strong-coupling theory for treatment. One method for realizing an experimental model of the system uses circularly-linked superconducting qubits.

The combination of Hubbard-type models with Jaynes-Cummings (atom-photon) interactions near the photon blockade regime originally appeared in three, roughly simultaneous papers in 2006.

All three papers explored systems of interacting atom-cavity systems, and shared much of the essential underlying physics. Nevertheless, the term Jaynes–Cummings–Hubbard was not coined until 2008.

Using mean-field theory to predict the phase diagram of the JCH model, the JCH model should exhibit Mott insulator and superfluid phases.

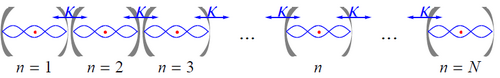
The Hamiltonian of the JCH model is (${\displaystyle \hbar =1}$):

${\displaystyle H=\sum _{n=1}^{N}\omega _{c}a_{n}^{\dagger }a_{n}+\sum _{n=1}^{N}\omega _{a}\sigma _{n}^{+}\sigma _{n}^{-}+\kappa \sum _{n=1}^{N}\left(a_{n+1}^{\dagger }a_{n}+a_{n}^{\dagger }a_{n+1}\right)+\eta \sum _{n=1}^{N}\left(a_{n}\sigma _{n}^{+}+a_{n}^{\dagger }\sigma _{n}^{-}\right)}$

where ${\displaystyle \sigma _{n}^{\pm }}$are Pauli operators for the two-level atom at the n-th cavity. The ${\displaystyle \kappa }$is the tunneling rate between neighboring cavities, and ${\displaystyle \eta }$is the vacuum Rabi frequency which characterizes to the photon-atom interaction strength. The cavity frequency is ${\displaystyle \omega _{c}}$and atomic transition frequency is ${\displaystyle \omega _{a}}$. The cavities are treated as periodic, so that the cavity labelled by n = N+1 corresponds to the cavity n = 1. Note that the model exhibits quantum tunneling; this process is similar to the Josephson effect.

Defining the photonic and atomic excitation number operators as ${\displaystyle {\hat {N}}_{c}\equiv \sum _{n=1}^{N}a_{n}^{\dagger }a_{n}}$and ${\displaystyle {\hat {N}}_{a}\equiv \sum _{n=1}^{N}\sigma _{n}^{+}\sigma _{n}^{-}}$, the total number of excitations is a conserved quantity, i.e., ${\displaystyle \lbrack H,{\hat {N}}_{c}+{\hat {N}}_{a}\rbrack =0}$.

The JCH Hamiltonian supports two-polariton bound states when the photon-atom interaction is sufficiently strong. In particular, the two polaritons associated with the bound states exhibit a strong correlation such that they stay close to each other in position space. This process is similar to the formation of a bound pair of repulsive bosonic atoms in an optical lattice.

• D. F. Walls and G. J. Milburn (1995), Quantum Optics, Springer-Verlag.